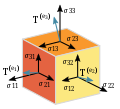
In tensor analysis, a mixed tensor is a tensor which is neither strictly covariant nor strictly contravariant; at least one of the indices of a mixed tensor...

In multilinear algebra, a tensor contraction is an operation on a tensor that arises from the canonical pairing of a vector space and its dual. In components...

two vectors is sometimes called an elementary tensor or a decomposable tensor. The elementary tensors span V ⊗ W {\displaystyle V\otimes W} in the sense...

electromagnetism, the electromagnetic tensor or electromagnetic field tensor (sometimes called the field strength tensor, Faraday tensor or Maxwell bivector) is a...

stress-energy tensor The stress–energy tensor, sometimes called the stress–energy–momentum tensor or the energy–momentum tensor, is a tensor field quantity...

(electromagnetic tensor, Maxwell tensor, permittivity, magnetic susceptibility, ...), and general relativity (stress–energy tensor, curvature tensor, ...). In...

differential geometry, a tensor density or relative tensor is a generalization of the tensor field concept. A tensor density transforms as a tensor field when passing...

of tensor theory. For expositions of tensor theory from different points of view, see: Tensor Tensor (intrinsic definition) Application of tensor theory...

In mathematics and physics, a tensor field is a function assigning a tensor to each point of a region of a mathematical space (typically a Euclidean space...

mathematics, the modern component-free approach to the theory of a tensor views a tensor as an abstract object, expressing some definite type of multilinear...

Riemann curvature tensor, the Weyl tensor expresses the tidal force that a body feels when moving along a geodesic. The Weyl tensor differs from the Riemann...

relationship between the Ricci tensor and the matter content of the universe. Like the metric tensor, the Ricci tensor assigns to each tangent space of...

Pseudovector Spinor Tensor Tensor algebra, Free algebra Tensor contraction Symmetric algebra, Symmetric power Symmetric tensor Mixed tensor Pandey, Divyanshu;...

notation and manipulation for tensors and tensor fields on a differentiable manifold, with or without a metric tensor or connection. It is also the modern...

mathematical field of differential geometry, the Riemann curvature tensor or Riemann–Christoffel tensor (after Bernhard Riemann and Elwin Bruno Christoffel) is the...

differential geometry, the Einstein tensor (named after Albert Einstein; also known as the trace-reversed Ricci tensor) is used to express the curvature...

metric field on M consists of a metric tensor at each point p of M that varies smoothly with p. A metric tensor g is positive-definite if g(v, v) > 0 for...

independent of any metric tensor and coordinate system. Also, the specific term "symbol" emphasizes that it is not a tensor because of how it transforms...

inertia tensor of a body calculated at its center of mass, and R {\displaystyle \mathbf {R} } be the displacement vector of the body. The inertia tensor of...

mathematics, the nonmetricity tensor in differential geometry is the covariant derivative of the metric tensor. It is therefore a tensor field of order three....

tensor is antisymmetric with respect to its first three indices. If a tensor changes sign under exchange of each pair of its indices, then the tensor...

notation Tensor definitions Tensor (intrinsic definition) Tensor field Tensor density Tensors in curvilinear coordinates Mixed tensor Antisymmetric tensor Symmetric...

thought of as a tensor, and is written δ j i {\displaystyle \delta _{j}^{i}} . Sometimes the Kronecker delta is called the substitution tensor. In the study...

relativity, a four-tensor is an abbreviation for a tensor in a four-dimensional spacetime. General four-tensors are usually written in tensor index notation...

alternating tensor subspace. On the other hand, the image A ( T ( V ) ) {\displaystyle {\mathcal {A}}(\mathrm {T} (V))} is always the alternating tensor graded...

differential geometry, the torsion tensor is a tensor that is associated to any affine connection. The torsion tensor is a bilinear map of two input vectors...

manifold M {\displaystyle M} and the metric tensor is given as a covariant, second-degree, symmetric tensor on M {\displaystyle M} , conventionally denoted...

fields) and to arbitrary tensor fields, in a unique way that ensures compatibility with the tensor product and trace operations (tensor contraction). Given...

In mathematics, a symmetric tensor is an unmixed tensor that is invariant under a permutation of its vector arguments: T ( v 1 , v 2 , … , v r ) = T (...

the tensor algebra of a vector space V, denoted T(V) or T•(V), is the algebra of tensors on V (of any rank) with multiplication being the tensor product...