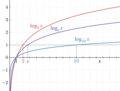
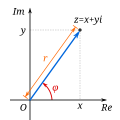
In mathematics, a complex logarithm is a generalization of the natural logarithm to nonzero complex numbers. The term refers to one of the following,...

the logarithm of a number is the exponent by which another fixed value, the base, must be raised to produce that number. For example, the logarithm of...

The natural logarithm of a number is its logarithm to the base of the mathematical constant e, which is an irrational and transcendental number approximately...

define the complex exponential from the complex logarithm. On the opposite, this is the complex logarithm that is often defined from the complex exponential...

both iπ and 3iπ are possible values for the complex logarithm of −1. In general, given any non-zero complex number w, any number z solving the equation...

for powers and logarithms for positive real numbers will fail for complex numbers, no matter how complex powers and complex logarithms are defined as...

the natural logarithm, i is the imaginary unit, and cos and sin are the trigonometric functions cosine and sine respectively. This complex exponential...

of the complex logarithm at the origin. Going once counterclockwise around a simple closed curve encircling the origin, the complex logarithm is incremented...

product logarithm, is a multivalued function, namely the branches of the converse relation of the function f(w) = wew, where w is any complex number and...

the logarithms of the terms. The complex logarithm is the complex number analogue of the logarithm function. No single valued function on the complex plane...

binary logarithm of 1 is 0, the binary logarithm of 2 is 1, the binary logarithm of 4 is 2, and the binary logarithm of 32 is 5. The binary logarithm is the...

multiple complex logarithms, and as a consequence of this, some matrices may have more than one logarithm, as explained below. If the matrix logarithm of B...

(}\exp(+iz)-\exp(-iz){\bigr )}} ⁠ (cf. Euler's formula). The principal branch of the complex logarithm function ⁠ log ⁡ z {\displaystyle \log z} ⁠ is holomorphic on the...

in this way, including the complex exponential function, complex logarithm functions, and trigonometric functions. Complex functions that are differentiable...

Binary logarithm Bode plot Henry Briggs Bygrave slide rule Cologarithm Common logarithm Complex logarithm Discrete logarithm Discrete logarithm records...

scalar will not affect the result. This is useful when one has the complex logarithm available. The extended argument of a number z (denoted as arg ¯ (...

look specifically at the exponential function, and the logarithm, as it is defined in complex analysis. The exponential function is single-valued, where...

All the familiar properties of the complex exponential function, the trigonometric functions, and the complex logarithm can be deduced directly from the...

the common logarithm (aka "standard logarithm") is the logarithm with base 10. It is also known as the decadic logarithm, the decimal logarithm and the Briggsian...

multivalued nature of complex power functions for non-integer exponents, one must be careful to specify the branch of the complex logarithm being used. In addition...

nonzero complex number has two square roots, three cube roots, and in general n nth roots. The only nth root of 0 is 0. The complex logarithm function...

constant approximately equal to 2.71828 that is the base of the natural logarithm and exponential function. It is sometimes called Euler's number, after...

{\displaystyle {\sqrt {4}}.} Consider the complex logarithm function log z. It is defined as the complex number w such that e w = z . {\displaystyle...

Most important are: power cepstrum: The logarithm is taken from the "power spectrum" complex cepstrum: The logarithm is taken from the spectrum, which is...

\pi } A similar problem appears with other complex functions with branch cuts, e.g., the complex logarithm and the relations logz + logw = log(zw) or...

multi-valued. Functions involving the complex logarithm typically inherit this property. Among these are the complex power, and, since zs appears in its...

U\subseteq \mathbb {C} \setminus \{0\}} the set of branches of the complex logarithm on U {\displaystyle U} . Given a point x {\displaystyle x} and an...

whole complex plane. However, it is meromorphic (even holomorphic) on C ∖ { 0 } {\displaystyle \mathbb {C} \setminus \{0\}} . The complex logarithm function...

mathematical notation for logarithms. All instances of log(x) without a subscript base should be interpreted as a natural logarithm, also commonly written...

the exponential is periodic, its inverse the complex logarithm is a multi-valued function, with each complex number in the domain corresponding to multiple...