mathematics, the inverse function of a function f (also called the inverse of f) is a function that undoes the operation of f. The inverse of f exists if...
43 KB (5,224 words) - 12:19, 12 March 2025
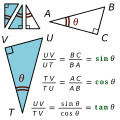
mathematics, the inverse trigonometric functions (occasionally also called antitrigonometric, cyclometric, or arcus functions) are the inverse functions of the...
75 KB (10,633 words) - 15:56, 30 April 2025
inverse function. The inverse function is also differentiable, and the inverse function rule expresses its derivative as the multiplicative inverse of...
42 KB (7,930 words) - 10:34, 27 April 2025
mathematics, the inverse hyperbolic functions are inverses of the hyperbolic functions, analogous to the inverse circular functions. There are six in...
27 KB (4,189 words) - 02:58, 22 April 2025
calculus, the inverse function rule is a formula that expresses the derivative of the inverse of a bijective and differentiable function f in terms of...
9 KB (1,789 words) - 13:15, 27 April 2025
interval I, it has an inverse function, which is a real function with domain f(I) and image I. This is how inverse trigonometric functions are defined in terms...
76 KB (11,411 words) - 13:49, 24 April 2025
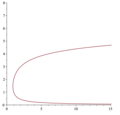
In mathematics, the inverse gamma function Γ − 1 ( x ) {\displaystyle \Gamma ^{-1}(x)} is the inverse function of the gamma function. In other words, y...
5 KB (815 words) - 07:05, 31 May 2024
percentile function (after the percentile), percent-point function, inverse cumulative distribution function (after the cumulative distribution function or c...
17 KB (2,174 words) - 18:12, 17 March 2025
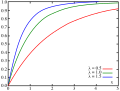
from any probability distribution given its cumulative distribution function. Inverse transformation sampling takes uniform samples of a number u {\displaystyle...
15 KB (2,085 words) - 23:32, 8 September 2024
mathematics, integrals of inverse functions can be computed by means of a formula that expresses the antiderivatives of the inverse f − 1 {\displaystyle f^{-1}}...
10 KB (1,696 words) - 03:08, 20 April 2025
The reciprocal function, the function f(x) that maps x to 1/x, is one of the simplest examples of a function which is its own inverse (an involution)...
15 KB (2,360 words) - 16:39, 28 November 2024
Involution (mathematics) (redirect from Self-inverse function)
mathematics, an involution, involutory function, or self-inverse function is a function f that is its own inverse, f(f(x)) = x for all x in the domain of...
17 KB (2,240 words) - 06:01, 19 February 2025
trigonometric functions has a corresponding inverse function, and an analog among the hyperbolic functions. The oldest definitions of trigonometric functions, related...
77 KB (10,740 words) - 04:00, 13 April 2025
considered above grows very rapidly, its inverse function, f−1, grows very slowly. This inverse Ackermann function f−1 is usually denoted by α. In fact,...
59 KB (7,095 words) - 10:07, 23 April 2025
the implicit function theorem. Inverse function theorem Constant rank theorem: Both the implicit function theorem and the inverse function theorem can...
22 KB (3,732 words) - 22:20, 24 April 2025
Image (mathematics) (redirect from Inverse image)
notion is rarely used. Image and inverse image may also be defined for general binary relations, not just functions. The word "image" is used in three...
19 KB (2,441 words) - 06:51, 3 April 2025
\end{aligned}}} The inverse of Φ is known as the normal quantile function, or probit function and may be expressed in terms of the inverse error function as probit...
47 KB (7,328 words) - 08:39, 27 April 2025
Sine and cosine (redirect from Sine function)
sine and cosine are not injective, their inverses are not exact inverse functions, but partial inverse functions. For example, sin ( 0 ) = 0 {\displaystyle...
56 KB (7,025 words) - 16:26, 27 March 2025
In economics, an inverse demand function is the mathematical relationship that expresses price as a function of quantity demanded (it is therefore also...
6 KB (943 words) - 15:29, 26 February 2025
real-valued function of a positive real variable, is the inverse function of the exponential function, leading to the identities: e ln x = x if x ∈ R +...
34 KB (5,882 words) - 17:23, 22 April 2025
F(x)=p} . This defines the inverse distribution function or quantile function. Some distributions do not have a unique inverse (for example if f X ( x )...
26 KB (3,993 words) - 17:49, 18 April 2025
Bijection (redirect from Bijective function)
integers to the even numbers, which has the division by two as its inverse function. A function is bijective if and only if it is both injective (or one-to-one)—meaning...
19 KB (2,509 words) - 18:58, 23 March 2025
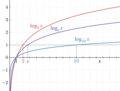
Logarithm (redirect from Logarithmic function)
written logb x, so log10 1000 = 3. As a single-variable function, the logarithm to base b is the inverse of exponentiation with base b. The logarithm base 10...
98 KB (11,674 words) - 22:46, 23 April 2025
implicit function is an inverse function. Not all functions have a unique inverse function. If g is a function of x that has a unique inverse, then the...
17 KB (2,204 words) - 03:08, 20 April 2025
Normal distribution (redirect from Normal density function)
/2}}}}} . The quantile function of a distribution is the inverse of the cumulative distribution function. The quantile function of the standard normal...
148 KB (22,625 words) - 14:53, 1 May 2025
trigonometric functions. The inverse hyperbolic functions are: inverse hyperbolic sine "arsinh" (also denoted "sinh−1", "asinh" or sometimes "arcsinh") inverse hyperbolic...
31 KB (4,989 words) - 21:48, 30 April 2025
domain. Every surjective function has a right inverse assuming the axiom of choice, and every function with a right inverse is necessarily a surjection...
18 KB (2,184 words) - 14:00, 10 January 2025
exponential function is the inverse function of the natural logarithm. The inverse function theorem implies that the natural logarithm has an inverse function, that...
37 KB (5,082 words) - 18:22, 10 April 2025
follows that the composition of two bijections is also a bijection. The inverse function of a composition (assumed invertible) has the property that (f ∘ g)−1...
37 KB (3,772 words) - 08:50, 25 February 2025
5^{2}(2-k^{2})+z^{2}-{}}}\cdots } The inverses of the Jacobi elliptic functions can be defined similarly to the inverse trigonometric functions; if x = sn ( ξ , m )...
73 KB (13,097 words) - 19:08, 2 March 2025