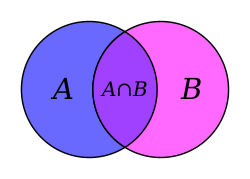
the axiom of power set is one of the Zermelo–Fraenkel axioms of axiomatic set theory. It guarantees for every set x {\displaystyle x} the existence of a...

theory of sets free of paradoxes such as Russell's paradox. Today, Zermelo–Fraenkel set theory, with the historically controversial axiom of choice (AC)...

example, in the ZFC axioms), the existence of the power set of any set is postulated by the axiom of power set. The powerset of S is variously denoted...

axiomatic set theory and the branches of logic, mathematics, and computer science that use it, the axiom of pairing is one of the axioms of Zermelo–Fraenkel...

axiomatic set theory and the branches of mathematics and philosophy that use it, the axiom of infinity is one of the axioms of Zermelo–Fraenkel set theory...

In set theory, the axiom schema of replacement is a schema of axioms in Zermelo–Fraenkel set theory (ZF) that asserts that the image of any set under any...

the whole of mathematics, particularly in the form of Zermelo–Fraenkel set theory with the axiom of choice. Besides its foundational role, set theory also...

union Axiom of infinity Axiom schema of replacement Axiom of power set Axiom of regularity Axiom schema of specification See also Zermelo set theory...

subclass of a set b {\displaystyle b} , so the axiom of separation implies ∪ a {\displaystyle \cup a} is a set. Likewise, the axiom of power set states...

The axiom of constructibility is a possible axiom for set theory in mathematics that asserts that every set is constructible. The axiom is usually written...

omitting the axioms Union, Power Set, Elementary Sets (essentially Pairing) and Infinity and then taking a theorem of Z, Adjunction, as an axiom. The natural...

of axiomatic set theory, the axiom schema of specification, also known as the axiom schema of separation (Aussonderungsaxiom), subset axiom, axiom of...

the axiom of regularity (also known as the axiom of foundation) is an axiom of Zermelo–Fraenkel set theory that states that every non-empty set A contains...

In set theory, the axiom of limitation of size was proposed by John von Neumann in his 1925 axiom system for sets and classes. It formalizes the limitation...

set theory, the axiom of union is one of the axioms of Zermelo–Fraenkel set theory. This axiom was introduced by Ernst Zermelo. Informally, the axiom...

The axiom of extensionality, also called the axiom of extent, is an axiom used in many forms of axiomatic set theory, such as Zermelo–Fraenkel set theory...

mathematics, the axiom of choice, abbreviated AC or AoC, is an axiom of set theory. Informally put, the axiom of choice says that given any collection of non-empty...

the unary predicate. AXIOM I. Axiom of extensionality (Axiom der Bestimmtheit) "If every element of a set M is also an element of N and vice versa ......

principle of excluded middle ( P E M {\displaystyle {\mathrm {PEM} }} ), constructive set theories often require some logical quantifiers in their axioms to...

inner model of ZF set theory (that is, of Zermelo–Fraenkel set theory with the axiom of choice excluded), and also that the axiom of choice and the generalized...

theorem does not rely on the axiom of choice. Cantor's theorem implies that no set is equinumerous to its power set (the set of all its subsets). This holds...

or the axiom of regularity and axiom of pairing. In Zermelo–Fraenkel set theory, the axiom of regularity and axiom of pairing prevent any set from containing...

non-conservative extension of Zermelo–Fraenkel set theory (ZFC) and is distinguished from other axiomatic set theories by the inclusion of Tarski's axiom, which states...

list of articles related to set theory. Algebra of sets Axiom of choice Axiom of countable choice Axiom of dependent choice Zorn's lemma Axiom of power set...

an axiom is a premise or starting point for reasoning. In mathematics, an axiom may be a "logical axiom" or a "non-logical axiom". Logical axioms are...

Axiom of pairing: If x, y are sets, then so is {x, y}, a set containing x and y as its only elements. Axiom of union: For any set x, there is a set y...

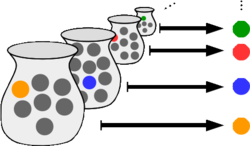
The axiom of countable choice or axiom of denumerable choice, denoted ACω, is an axiom of set theory that states that every countable collection of non-empty...

the power set operator. Therefore, the existence of the Cartesian product of any two sets in ZFC follows from the axioms of pairing, union, power set, and...

Dedekind-finite set is also finite, but this implication cannot be proved in ZF (Zermelo–Fraenkel axioms without the axiom of choice) alone. The axiom of countable...

non-well-founded set theories, the foundation axiom of ZFC is replaced by axioms implying its negation. The study of non-well-founded sets was initiated...