The binomial sum variance inequality states that the variance of the sum of binomially distributed random variables will always be less than or equal to...
7 KB (1,439 words) - 06:32, 14 April 2025
Chebyshev–Markov–Stieltjes inequalities Chebyshev's sum inequality Clarkson's inequalities Eilenberg's inequality Fekete–Szegő inequality Fenchel's inequality Friedrichs's...
9 KB (709 words) - 21:10, 14 April 2025
interval concepts, Michael Short has shown that inequalities on the approximation error between the binomial distribution and the normal distribution can...
42 KB (6,213 words) - 23:11, 12 May 2025
probability p, then the variance of the sum will be smaller than the variance of a binomial variable distributed as B(n + m, p). The binomial distribution is...
53 KB (7,554 words) - 05:20, 9 January 2025
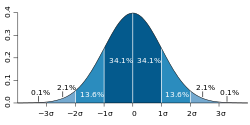
Normal distribution (section Zero-variance limit)
for example: The binomial distribution B ( n , p ) {\textstyle B(n,p)} is approximately normal with mean n p {\textstyle np} and variance n p ( 1 − p ) {\textstyle...
148 KB (22,607 words) - 17:11, 14 May 2025
study of the contributions to sums of squares. Laplace knew how to estimate a variance from a residual (rather than a total) sum of squares. By 1827, Laplace...
56 KB (7,645 words) - 21:36, 7 April 2025
Median (redirect from Variance of the median)
the minimum-variance mean (for large normal samples), which is to say the variance of the median will be ~50% greater than the variance of the mean....
63 KB (8,022 words) - 22:05, 30 April 2025
Poisson distribution (category Factorial and binomial topics)
unlikely to make a call to that switchboard in that hour. The variance of the binomial distribution is 1 − p times that of the Poisson distribution, so...
81 KB (11,215 words) - 08:39, 14 May 2025
distribution, the distribution of a sum of squared standard normal variables; useful e.g. for inference regarding the sample variance of normally distributed samples...
48 KB (6,688 words) - 17:43, 6 May 2025
Coefficient of variation (redirect from Coefficient of Variance)
distribution) are considered low-variance, while those with CV > 1 (such as a hyper-exponential distribution) are considered high-variance[citation needed]. Some...
30 KB (4,017 words) - 13:36, 17 April 2025
the events happens is no greater than the sum of the probabilities of the individual events. This inequality provides an upper bound on the probability...
10 KB (1,945 words) - 15:49, 24 March 2025
Mann–Whitney U test (redirect from Wilcoxon rank-sum test)
\over 12}-{n_{1}n_{2}\sum _{k=1}^{K}(t_{k}^{3}-t_{k}) \over 12n(n-1)}}},\,} where the left side is simply the variance and the right side is the adjustment...
44 KB (5,746 words) - 20:16, 8 April 2025
example, the variance of a sum of uncorrelated random variables is equal to the sum of their variances. A disadvantage of the variance for practical...
61 KB (10,215 words) - 11:05, 7 May 2025
finite variance σ 2 {\textstyle \sigma ^{2}} . The sum X 1 + ⋯ + X n {\textstyle X_{1}+\cdots +X_{n}} has mean n μ {\textstyle n\mu } and variance n σ 2...
67 KB (9,171 words) - 16:52, 28 April 2025
Covariance (redirect from Co-variance)
the sample space. As a result, for random variables with finite variance, the inequality | cov ( X , Y ) | ≤ σ 2 ( X ) σ 2 ( Y ) {\displaystyle \left|\operatorname...
29 KB (4,754 words) - 01:56, 4 May 2025
Expected value (section Inequalities)
{\frac {\operatorname {Var} [X]}{a^{2}}},} where Var is the variance. These inequalities are significant for their nearly complete lack of conditional...
52 KB (7,614 words) - 17:41, 4 May 2025
The variance of the sum is equal to the sum of the variances, which is asymptotic to n 2 / log n {\displaystyle n^{2}/\log n} . The variance of the...
45 KB (6,398 words) - 10:46, 8 May 2025
Standard deviation (redirect from Standard variance)
rule Accuracy and precision Algorithms for calculating variance Chebyshev's inequality An inequality on location and scale parameters Coefficient of variation...
59 KB (8,233 words) - 19:16, 23 April 2025
Bernoulli distribution (section Variance)
then their sum is distributed according to a binomial distribution with parameters n and p: ∑ k = 1 n X k ∼ B ( n , p ) {\displaystyle \sum _{k=1}^{n}X_{k}\sim...
13 KB (2,196 words) - 21:53, 27 April 2025
E (mathematical constant) (section Inequalities)
characterizations using the limit and the infinite series can be proved via the binomial theorem. Jacob Bernoulli discovered this constant in 1683, while studying...
54 KB (6,480 words) - 19:11, 22 April 2025
Kurtosis (section Variance under normality)
For non-normal samples, the variance of the sample variance depends on the kurtosis; for details, please see variance. Pearson's definition of kurtosis...
37 KB (5,310 words) - 21:10, 14 April 2025
{1}{\bar {x}}}\right)={\frac {n-2}{\sum _{i}x_{i}}}} This is derived from the mean and variance of the inverse-gamma distribution, Inv-Gamma...
43 KB (6,647 words) - 17:34, 15 April 2025
Cauchy distribution (category Probability distributions with non-finite variance)
large numbers for any weighted sum of independent Cauchy distributions. This shows that the condition of finite variance in the central limit theorem cannot...
47 KB (6,933 words) - 19:26, 1 April 2025
Covariance matrix (redirect from Variance-covariance matrix)
matrix (also known as auto-covariance matrix, dispersion matrix, variance matrix, or variance–covariance matrix) is a square matrix giving the covariance between...
37 KB (5,799 words) - 21:03, 14 April 2025
Wald test Bernstein inequalities (probability theory) Binomial regression Binomial proportion confidence interval Chebyshev's inequality Chernoff bound Gauss's...
3 KB (279 words) - 13:03, 9 April 2024
Beta distribution (category Factorial and binomial topics)
)=F_{\text{binomial}}(\beta -1;\alpha +\beta -1,1-x)} . The beta distribution may also be reparameterized in terms of its mean μ (0 < μ < 1) and the sum of the...
245 KB (40,562 words) - 12:56, 14 May 2025
Sub-Gaussian distribution (section Variance proxy)
distribution is strictly subgaussian, any symmetric Binomial distribution is strictly subgaussian. The optimal variance proxy ‖ X ‖ v p 2 {\displaystyle \Vert X\Vert...
36 KB (7,008 words) - 16:28, 3 March 2025
Fisher information (section Isoperimetric inequality)
. Since the estimator is unbiased, its MSE equals its variance. By rearranging, the inequality tells us that Var ( θ ^ ) ≥ 1 I ( θ ) . {\displaystyle...
52 KB (7,377 words) - 23:47, 17 April 2025
Ratio estimator (section Variance estimates)
{y}}{\bar {x}}}={\frac {\sum _{i=1}^{n}y_{i}}{\sum _{i=1}^{n}x_{i}}}} That the ratio is biased can be shown with Jensen's inequality as follows (assuming...
22 KB (4,015 words) - 19:09, 2 May 2025
the mean may be derived from the variance of a sum of independent random variables, given the definition of variance and some properties thereof. If x...
20 KB (2,781 words) - 03:46, 4 May 2025