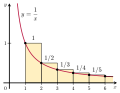
In mathematics, the integral test for convergence is a method used to test infinite series of monotonic terms for convergence. It was developed by Colin...

improper integral converges or diverges by comparing the series or integral to one whose convergence properties are known. In calculus, the comparison test for...

test is a necessary and sufficient condition for convergence due to the non-Archimedean ultrametric triangle inequality. Unlike stronger convergence tests...

mathematics, the Cauchy condensation test, named after Augustin-Louis Cauchy, is a standard convergence test for infinite series. For a non-increasing sequence f...

test for the convergence of infinite series. It can also be proven to diverge by comparing the sum to an integral, according to the integral test for...

mathematics, convergence tests are methods of testing for the convergence, conditional convergence, absolute convergence, interval of convergence or divergence...

Dirichlet's test is a method of testing for the convergence of a series that is especially useful for proving conditional convergence. It is named after...

In mathematics, the root test is a criterion for the convergence (a convergence test) of an infinite series. It depends on the quantity lim sup n → ∞...

Cauchy's test may refer to: Cauchy's root test Cauchy's condensation test the integral test for convergence, sometimes known as the Maclaurin–Cauchy test These...

by parts. A closely related convergence test, also known as Abel's test, can often be used to establish the convergence of a power series on the boundary...

easily calculated and evaluated for convergence directly, convergence tests can be used to prove that the series converges or diverges. In ordinary finite...

convergence, Uniform convergence Absolute convergence, Conditional convergence Normal convergence Radius of convergence Integral test for convergence...

Comparison test can mean: Limit comparison test, a method of testing for the convergence of an infinite series. Direct comparison test, a way of deducing...

the limit comparison test (LCT) (in contrast with the related direct comparison test) is a method of testing for the convergence of an infinite series...

Many standard tests for divergence and convergence, most notably including the ratio test and the root test, demonstrate absolute convergence. This is because...

mathematical field of analysis, uniform convergence is a mode of convergence of functions stronger than pointwise convergence. A sequence of functions ( f n )...

{1}{j}}} This can be estimated using the integral test for convergence, or more directly by comparing it to the integral of 1 / x {\displaystyle 1/x} from k...

In mathematics, the ratio test is a test (or "criterion") for the convergence of a series ∑ n = 1 ∞ a n , {\displaystyle \sum _{n=1}^{\infty }a_{n},}...

unconditional convergence." ... "In fact, for improper integrals of such functions, unconditional convergence turns out to be equivalent to absolute convergence."...

_{n=6}^{\infty }{\frac {1}{2n\log n}}=\infty \end{aligned}}} by the integral test for convergence. This shows that the series on the left diverges. The following...

the Leibniz integral rule for differentiation under the integral sign, named after Gottfried Wilhelm Leibniz, states that for an integral of the form...

that for integrals on unbounded intervals, uniform convergence of a function is not strong enough to allow passing a limit through an integral sign....

difficult to compute for commonly seen types of series. Integral test. The series can be compared to an integral to establish convergence or divergence. Let...

many conditions for the convergence of Fourier series. The original test was established by Peter Gustav Lejeune Dirichlet in 1829, for piecewise monotone...

there are various notions of the convergence of measures. For an intuitive general sense of what is meant by convergence of measures, consider a sequence...

Riemann integral or the generalized Riemann or Henstock–Kurzweil integral. This can be seen by using Dirichlet's test for improper integrals. It is a...

be rearranged to create arbitrary convergence. Agnew's theorem describes rearrangements that preserve convergence for all convergent series. The general...

integral, and curvilinear integral are also used; contour integral is used as well, although that is typically reserved for line integrals in the complex plane...

limits under the integral sign (via the monotone convergence theorem and dominated convergence theorem). While the Riemann integral considers the area...

Integration is the basic operation in integral calculus. While differentiation has straightforward rules by which the derivative of a complicated function...