orientation of a real vector bundle is a generalization of an orientation of a vector space; thus, given a real vector bundle π: E →B, an orientation...

take two copies of M {\displaystyle M} , each of which corresponds to a different orientation. A real vector bundle, which a priori has a GL(n) structure...

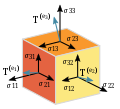
The orientation of a real vector space or simply orientation of a vector space is the arbitrary choice of which ordered bases are "positively" oriented...

bundle. A sphere bundle that is a product space is orientable, as is any sphere bundle over a simply connected space. If E be a real vector bundle on a space...

class is a characteristic class of oriented, real vector bundles. Like other characteristic classes, it measures how "twisted" the vector bundle is. In...

characteristic classes associated with complex vector bundles. They have since become fundamental concepts in many branches of mathematics and physics, such as string...

Thom and Lev Pontryagin) of algebraic topology and differential topology is a topological space associated to a vector bundle, over any paracompact space...

made, since the density bundle is the tensor product of the orientation bundle of M and the n-th exterior product bundle of T∗M (see pseudotensor). In...

In mathematics, a symplectic vector space is a vector space V {\displaystyle V} over a field F {\displaystyle F} (for example the real numbers R {\displaystyle...

of the ambient space minus the dimension of the submanifold. Connected sum Connection Cotangent bundle – the vector bundle of cotangent spaces on a manifold...

In mathematics, a vector bundle is said to be flat if it is endowed with a linear connection with vanishing curvature, i.e. a flat connection. Let π :...

physics, and engineering, a Euclidean vector or simply a vector (sometimes called a geometric vector or spatial vector) is a geometric object that has...

group of the frame bundle to G {\displaystyle G} . The following examples are defined for real vector bundles, particularly the tangent bundle of a smooth...

algebra. A vector bundle is a family of vector spaces parametrized continuously by a topological space X. More precisely, a vector bundle over X is a topological...

mathematics, a tensor is an algebraic object that describes a multilinear relationship between sets of algebraic objects related to a vector space. Tensors...

{R} } defines a linear functional on each tangent space of M, and therefore it factors through the trivial bundle M × R. The vector bundle morphism ⋀ k...

products of the tangent bundle and the cotangent bundle. Each element of the bundle is a tensor field, which can act as a multilinear operator on vector fields...

physics, gauge theory is the general study of connections on vector bundles, principal bundles, and fibre bundles. Gauge theory in mathematics should not...

straightforwardly to a notion of differentiation associated to a connection on a vector bundle, also known as a Koszul connection. Historically, at the turn of the 20th...

mathematics, a metric connection is a connection in a vector bundle E equipped with a bundle metric; that is, a metric for which the inner product of any two...

roots" of vectors (although this is inaccurate and may be misleading; they are better viewed as "square roots" of sections of vector bundles – in the...

point. A normal vector is a vector perpendicular to a given object at a particular point. A normal vector of length one is called a unit normal vector or...

Grassmann algebra of a vector space V {\displaystyle V} is an associative algebra that contains V , {\displaystyle V,} which has a product, called exterior...

mathematics, a set B of elements of a vector space V is called a basis (pl.: bases) if every element of V can be written in a unique way as a finite linear...

In vector calculus, the curl, also known as rotor, is a vector operator that describes the infinitesimal circulation of a vector field in three-dimensional...

fibration (also known as the Hopf bundle or Hopf map) describes a 3-sphere (a hypersphere in four-dimensional space) in terms of circles and an ordinary sphere...

varieties, proved by Jean-Pierre Serre. The basic version applies to vector bundles on a smooth projective variety, but Alexander Grothendieck found wide...

a principal G L + ( n ) {\displaystyle \mathrm {GL} ^{+}(n)} sub-bundle of the linear frame bundle of M , {\displaystyle M,} and so the orientation associated...

π0(KO) is a vector bundle over S0, which consists of two points. Thus over each point, the bundle is trivial, and the non-triviality of the bundle is the...

operator or Hodge star is a linear map defined on the exterior algebra of a finite-dimensional oriented vector space endowed with a nondegenerate symmetric...