In mathematics, a set is a collection of different things; these things are called elements or members of the set and are typically mathematical objects...
49 KB (7,058 words) - 19:56, 18 May 2025
a set, set theory – as a branch of mathematics – is mostly concerned with those that are relevant to mathematics as a whole. The modern study of set theory...
54 KB (6,575 words) - 12:01, 1 May 2025
In mathematics, more specifically in point-set topology, the derived set of a subset S {\displaystyle S} of a topological space is the set of all limit...
11 KB (1,544 words) - 19:40, 9 April 2025
mathematics, the algebra of sets, not to be confused with the mathematical structure of an algebra of sets, defines the properties and laws of sets,...
15 KB (1,865 words) - 16:28, 28 May 2024
any of some sets "Complement and Set Difference". web.mnstate.edu. Retrieved 2020-09-04. "Complement (set) Definition (Illustrated Mathematics Dictionary)"...
12 KB (1,515 words) - 07:59, 27 January 2025
empty set. For explanation of the symbols used in this article, refer to the table of mathematical symbols. The union of two sets A and B is the set of elements...
14 KB (1,989 words) - 08:46, 6 May 2025
logic Set theory – Branch of mathematics that studies sets Number theory – Branch of mathematics Combinatorics – Branch of discrete mathematics Finite...
10 KB (1,824 words) - 18:20, 19 February 2025
Subset (redirect from Superset (mathematics))
In mathematics, a set A is a subset of a set B if all elements of A are also elements of B; B is then a superset of A. It is possible for A and B to be...
11 KB (1,734 words) - 18:05, 12 March 2025
In mathematics, the empty set or void set is the unique set having no elements; its size or cardinality (count of elements in a set) is zero. Some axiomatic...
15 KB (2,229 words) - 16:03, 21 April 2025
In mathematics, and particularly in set theory, category theory, type theory, and the foundations of mathematics, a universe is a collection that contains...
18 KB (2,649 words) - 04:29, 23 August 2024
In mathematics, an element (or member) of a set is any one of the distinct objects that belong to that set. For example, given a set called A containing...
7 KB (846 words) - 03:34, 23 March 2025
Naive set theory is any of several theories of sets used in the discussion of the foundations of mathematics. Unlike axiomatic set theories, which are...
34 KB (4,716 words) - 15:17, 3 April 2025
(the study of continuous changes), and set theory (presently used as a foundation for all mathematics). Mathematics involves the description and manipulation...
163 KB (15,937 words) - 09:11, 18 May 2025
Equivalence class (redirect from Class representative (mathematics))
In mathematics, when the elements of some set S {\displaystyle S} have a notion of equivalence (formalized as an equivalence relation), then one may naturally...
18 KB (2,700 words) - 04:06, 2 May 2025
The History of Mathematics: An Introduction include how to define "elements" or parts of a set, how to define unique elements in the set, and how to prove...
8 KB (917 words) - 03:24, 10 May 2025
formulas. Commonly encountered mathematical objects include numbers, expressions, shapes, functions, and sets. Mathematical objects can be very complex;...
27 KB (2,770 words) - 17:40, 5 May 2025
In mathematics, a singleton (also known as a unit set or one-point set) is a set with exactly one element. For example, the set { 0 } {\displaystyle \{0\}}...
6 KB (828 words) - 18:55, 11 May 2025
examines the implementation of mathematical concepts in set theory. The implementation of a number of basic mathematical concepts is carried out in parallel...
59 KB (10,960 words) - 22:58, 2 May 2025
because it is not itself a set. Universe (mathematics) Grothendieck universe Domain of discourse Von Neumann–Bernays–Gödel set theory — an extension of...
10 KB (1,327 words) - 06:43, 21 May 2024
bijection with the set of natural numbers) rather than "continuous" (analogously to continuous functions). Objects studied in discrete mathematics include integers...
26 KB (2,771 words) - 14:34, 10 May 2025
In mathematics, an uncountable set, informally, is an infinite set that contains too many elements to be countable. The uncountability of a set is closely...
6 KB (884 words) - 06:49, 8 April 2025
Mathematical logic is the study of formal logic within mathematics. Major subareas include model theory, proof theory, set theory, and recursion theory...
69 KB (8,370 words) - 19:50, 19 April 2025
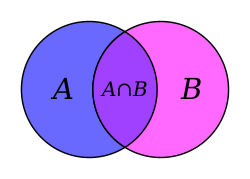
Venn diagram (redirect from Set diagram)
combinatorial mathematics. Boston: Addison-Wesley. p. 143. ISBN 978-0-201-72634-3. Johnson, David L. (2001). "3.3 Laws". Elements of logic via numbers and sets. Springer...
31 KB (3,242 words) - 13:31, 22 April 2025
symbols used in this article, refer to the table of mathematical symbols. The intersection of two sets A {\displaystyle A} and B , {\displaystyle B,} denoted...
12 KB (1,732 words) - 23:16, 26 December 2023
the standard form of axiomatic set theory and as such is the most common foundation of mathematics. Zermelo–Fraenkel set theory with the axiom of choice...
46 KB (6,252 words) - 14:45, 16 April 2025
In mathematics, the power set (or powerset) of a set S is the set of all subsets of S, including the empty set and S itself. In axiomatic set theory (as...
21 KB (2,479 words) - 08:13, 23 April 2025
set theory and related branches of mathematics, a family (or collection) can mean, depending upon the context, any of the following: set, indexed set...
10 KB (1,533 words) - 02:20, 8 February 2025
In mathematics, a set is countable if either it is finite or it can be made in one to one correspondence with the set of natural numbers. Equivalently...
28 KB (4,381 words) - 01:01, 29 March 2025
equivalent over ZF set theory. The goal of reverse mathematics, however, is to study possible axioms of ordinary theorems of mathematics rather than possible...
38 KB (4,781 words) - 18:58, 11 April 2025
{Z} ,n=2k\}} The set of all even integers, expressed in set-builder notation. In mathematics and more specifically in set theory, set-builder notation...
14 KB (1,917 words) - 20:19, 4 March 2025