the tensor product of two fields is their tensor product as algebras over a common subfield. If no subfield is explicitly specified, the two fields must...

v\otimes w} is called the tensor product of v and w. An element of V ⊗ W {\displaystyle V\otimes W} is a tensor, and the tensor product of two vectors is sometimes...

the shorter form "tensor". For example, the Riemann curvature tensor refers a tensor field, as it associates a tensor to each point of a Riemannian manifold...

glossary of tensor theory. For expositions of tensor theory from different points of view, see: Tensor Tensor (intrinsic definition) Application of tensor theory...

the tensor product of two algebras over a commutative ring R is also an R-algebra. This gives the tensor product of algebras. When the ring is a field, the...

of differential operators. Tor functor Tensor product of algebras Tensor product of fields Derived tensor product Eilenberg–Watts theorem Tensoring with...

over a field (or other commutative ring) Tensor product of representations, a special case in representation theory Tensor product of fields, an operation...

geometry, a tensor density or relative tensor is a generalization of the tensor field concept. A tensor density transforms as a tensor field when passing...

(stress–energy tensor, curvature tensor, ...). In applications, it is common to study situations in which a different tensor can occur at each point of an object;...

component-free approach to the theory of a tensor views a tensor as an abstract object, expressing some definite type of multilinear concept. Their properties...

A metric tensor g is positive-definite if g(v, v) > 0 for every nonzero vector v. A manifold equipped with a positive-definite metric tensor is known...

In multilinear algebra, a tensor contraction is an operation on a tensor that arises from the canonical pairing of a vector space and its dual. In components...

stress-energy tensor The stress–energy tensor, sometimes called the stress–energy–momentum tensor or the energy–momentum tensor, is a tensor physical quantity...

electromagnetism, the electromagnetic tensor or electromagnetic field tensor (sometimes called the field strength tensor, Faraday tensor or Maxwell bivector) is a...

In multilinear algebra, a tensor decomposition is any scheme for expressing a "data tensor" (M-way array) as a sequence of elementary operations acting...

of a common field then the (external) composite is defined using the tensor product of fields. Note that some care has to be taken for the choice of the...

number of real roots and 2r2 is the number of non-real complex roots of f (which come in complex conjugate pairs); write the tensor product of fields K ⊗...

constitutes the rules of index notation and manipulation for tensors and tensor fields on a differentiable manifold, with or without a metric tensor or connection...

learning, the term tensor informally refers to two different concepts (i) a way of organizing data and (ii) a multilinear (tensor) transformation. Data...

perfect. That finite fields are perfect follows a posteriori from their known structure. One can show that the tensor product of fields of L with itself over...

gluon field strength tensor is a second order tensor field characterizing the gluon interaction between quarks. The strong interaction is one of the fundamental...

product, sometimes denoted by ⊗, is an operation on two matrices of arbitrary size resulting in a block matrix. It is a specialization of the tensor product...

In the mathematical field of differential geometry, the Riemann curvature tensor or Riemann–Christoffel tensor (after Bernhard Riemann and Elwin Bruno...

of which one can recognise categories of G-sets for G profinite. To see how this applies to the case of fields, one has to study the tensor product of...

a Cartesian tensor uses an orthonormal basis to represent a tensor in a Euclidean space in the form of components. Converting a tensor's components from...

mathematics, specifically multilinear algebra, a dyadic or dyadic tensor is a second order tensor, written in a notation that fits in with vector algebra. There...

In mathematics, the tensor product of representations is a tensor product of vector spaces underlying representations together with the factor-wise group...

indeterminates ai, E is any field, and n ≥ 5). The tensor product of fields is not usually a field. For example, a finite extension F / E of degree n is a Galois...

reduced rings Dual numbers Tensor product of fields Tensor product of R-algebras Quotient ring Field of fractions Product of rings Annihilator (ring theory)...

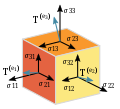
In mathematics, a symmetric tensor is an unmixed tensor that is invariant under a permutation of its vector arguments: T ( v 1 , v 2 , … , v r ) = T (...