if n is an odd integer. Even functions are those real functions whose graph is self-symmetric with respect to the y-axis, and odd functions are those whose...

Examples of odd functions are x, x3, sin(x), sinh(x), and erf(x). The integral of an odd function from −A to +A is zero, provided that A is finite and that the...

integer arithmetic. even ± even = even; even ± odd = odd; odd ± odd = even; even × even = even; even × odd = even; odd × odd = odd; By construction in...

Fourier sine and cosine transforms are integral equations that decompose arbitrary functions into a sum of sine waves representing the odd component of...

shares many characteristics. It functions by comparing all odd/even indexed pairs of adjacent elements in the list and swapping pairs where in the wrong...

V.} Symmetric functions should not be confused with even and odd functions, which have a different sort of symmetry. Given any function f {\displaystyle...

bias and bias toward/away from zero, provided the numbers to be rounded are neither mostly even nor mostly odd. It also shares the round half to even property...

permutations of X (i.e. the bijective functions from X to X) fall into two classes of equal size: the even permutations and the odd permutations. If any total ordering...

an integer is even if dividing by two yields an integer even and odd functions, a function is even if f(−x) = f(x) for all x even and odd permutations...

of ones Parity learning, a problem in machine learning Parity of even and odd functions Parity (physics), a symmetry property of physical quantities or...

also refer to: Even and odd numbers, an integer is odd if dividing by two does not yield an integer Even and odd functions, a function is odd if f(−x) = −f(x)...

Examples include even and odd functions in calculus, symmetric groups in abstract algebra, symmetric matrices in linear algebra, and Galois groups in...

parity—the quality of an integer being even or odd—is even. This can be easily verified based on the definition of "even": zero is an integer multiple of 2...

all arguments. The even and odd functions satisfy by definition simple reflection relations around a = 0. For all even functions, f ( − x ) = f ( x )...

the string is even or odd. Accordingly, there are two variants of parity bits: even parity bit and odd parity bit. In the case of even parity, for a given...

{\displaystyle -\beta } for β {\displaystyle \beta } and using the reflection identities of even and odd functions, we also get: sin ⁡ ( α − β ) = sin ⁡ α cos...

g=g\star f} . Complex conjugate – Fundamental operation on complex numbers Even and odd functions – Functions such that f(–x) equals f(x) or –f(x) v t e...

Even and odd functions Real and imaginary parts Jones, Frank (2001). Lebesgue integration on Euclidean space (Rev. ed.). Sudbury, MA: Jones and Bartlett...

exponential functions, logarithmic functions, floor function, fractional part function, signum function, even and odd functions, periodic functions, composite...

Unsolved problem in mathematics For even numbers, divide by 2; For odd numbers, multiply by 3 and add 1. With enough repetition, do all positive integers...

In mathematics, hyperbolic functions are analogues of the ordinary trigonometric functions, but defined using the hyperbola rather than the circle. Just...

generate even harmonics or an odd function for odd harmonics. See Even and odd functions#Harmonics. A full wave rectifier, for example, is good for making...

proved that all even perfect numbers are of this form. This is known as the Euclid–Euler theorem. It is not known whether there are any odd perfect numbers...

In mathematics, Mathieu functions, sometimes called angular Mathieu functions, are solutions of Mathieu's differential equation d 2 y d x 2 + ( a − 2...

the function value must cross zero, in the process of changing from negative to positive or vice versa (which always happens for odd functions). The...

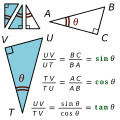
mathematics, the trigonometric functions (also called circular functions, angle functions or goniometric functions) are real functions which relate an angle of...

the Rodrigues formulae given above, we can see that Hn(x) and Hen(x) are even or odd functions depending on n: H n ( − x ) = ( − 1 ) n H n ( x ) , He n...

prime-counting functions. This article provides links to functions of both classes. An example of an arithmetic function is the divisor function whose value...

/\partial y} are odd functions, where ∂ / ∂ ξ j {\displaystyle \partial /\partial \xi _{j}} again mean right derivatives. When the function D {\displaystyle...

data with odd symmetry (since the Fourier transform of a real and odd function is imaginary and odd), where in some variants the input and/or output data...