In the mathematical field of differential geometry, a metric tensor (or simply metric) is an additional structure on a manifold M (such as a surface)...

differentiable manifold M {\displaystyle M} and the metric tensor is given as a covariant, second-degree, symmetric tensor on M {\displaystyle M} , conventionally...

Minkowski metric η is the metric tensor of Minkowski space. It is a pseudo-Euclidean metric, or more generally, a constant pseudo-Riemannian metric in Cartesian...

the Einstein tensor, g μ ν {\displaystyle g_{\mu \nu }} is the metric tensor, T μ ν {\displaystyle T_{\mu \nu }} is the stress–energy tensor, Λ {\displaystyle...

^{*}M} of a Riemannian or pseudo-Riemannian manifold induced by its metric tensor. There are similar isomorphisms on symplectic manifolds. The term musical...

(electromagnetic tensor, Maxwell tensor, permittivity, magnetic susceptibility, ...), and general relativity (stress–energy tensor, curvature tensor, ...). In...

the Riemann tensor. This tensor has the same symmetries as the Riemann tensor, but satisfies the extra condition that it is trace-free: metric contraction...

geometry of a given metric tensor differs locally from that of ordinary Euclidean space or pseudo-Euclidean space. The Ricci tensor can be characterized...

In multilinear algebra, a tensor contraction is an operation on a tensor that arises from the canonical pairing of a vector space and its dual. In components...

called a semi-Riemannian manifold, is a differentiable manifold with a metric tensor that is everywhere nondegenerate. This is a generalization of a Riemannian...

differential geometry, the Einstein tensor (named after Albert Einstein; also known as the trace-reversed Ricci tensor) is used to express the curvature...

notation and manipulation for tensors and tensor fields on a differentiable manifold, with or without a metric tensor or connection. It is also the modern...

In tensor analysis, a mixed tensor is a tensor which is neither strictly covariant nor strictly contravariant; at least one of the indices of a mixed...

In mathematics, the signature of a metric tensor g (or equivalently, a real quadratic form thought of as a real symmetric bilinear form on a finite-dimensional...

In mathematics, the Poincaré metric, named after Henri Poincaré, is the metric tensor describing a two-dimensional surface of constant negative curvature...

mathematics, the modern component-free approach to the theory of a tensor views a tensor as an abstract object, expressing some definite type of multilinear...

stress-energy tensor The stress–energy tensor, sometimes called the stress–energy–momentum tensor or the energy–momentum tensor, is a tensor physical quantity...

mathematics, the nonmetricity tensor in differential geometry is the covariant derivative of the metric tensor. It is therefore a tensor field of order three....

energy–momentum tensor and the Petrov classification of the Weyl tensor. There are various methods of classifying these tensors, some of which use tensor invariants...

are independent of any metric tensor and coordinate system. Also, the specific term "symbol" emphasizes that it is not a tensor because of how it transforms...

It assigns a tensor to each point of a Riemannian manifold (i.e., it is a tensor field). It is a local invariant of Riemannian metrics that measures...

metric (FLRW), where it corresponds to an increase in the scale of the spatial part of the universe's spacetime metric tensor (which governs...

by how the tangent space is attached to the cotangent space by the metric tensor. Abstractly, one would say that the manifold has an associated (orthonormal)...

of tensor theory. For expositions of tensor theory from different points of view, see: Tensor Tensor (intrinsic definition) Application of tensor theory...

the field strength tensor, a classical one using R as the curvature tensor, and the classical notation for the Riemann curvature tensor, most of which can...

through the metric tensor, which is a tensor field with one tensor at each point of the space-time manifold, and each belonging to the tensor product of...

theoretical physics, the induced metric is the metric tensor defined on a submanifold that is induced from the metric tensor on a manifold into which the...

Alternatively, the metric tensor can be specified by writing down a coframe in terms of a coordinate basis and stipulating that the metric tensor is given by...

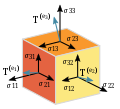
In mathematics, a symmetric tensor is an unmixed tensor that is invariant under a permutation of its vector arguments: T ( v 1 , v 2 , … , v r ) = T (...

differential geometry, a tensor density or relative tensor is a generalization of the tensor field concept. A tensor density transforms as a tensor field when passing...