The Riemann zeta function or Euler–Riemann zeta function, denoted by the Greek letter ζ (zeta), is a mathematical function of a complex variable defined...

Riemann zeta function is a function in complex analysis, which is also important in number theory. It is often denoted ζ ( s ) {\displaystyle \zeta (s)}...

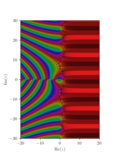
non-trivial zeroes of the Riemann zeta function have a real part of one half? More unsolved problems in mathematics In mathematics, the Riemann hypothesis is the...

the Dedekind zeta function of an algebraic number field K, generally denoted ζK(s), is a generalization of the Riemann zeta function (which is obtained...

L-function via analytic continuation. The Riemann zeta function is an example of an L-function, and some important conjectures involving L-functions are...

universality of zeta functions is the remarkable ability of the Riemann zeta function and other similar functions (such as the Dirichlet L-functions) to approximate...

{1}{2}}\vartheta (0;\tau )} was used by Riemann to prove the functional equation for the Riemann zeta function, by means of the Mellin transform Γ ( s...

by Bernhard Riemann in his seminal 1859 paper "On the Number of Primes Less Than a Given Magnitude", in which he defined his zeta function and proved its...

Riemann xi function is a variant of the Riemann zeta function, and is defined so as to have a particularly simple functional equation. The function is...

The Riemann hypothesis is one of the most important conjectures in mathematics. It is a statement about the zeros of the Riemann zeta function. Various...

arithmetic zeta function is a zeta function associated with a scheme of finite type over integers. The arithmetic zeta function generalizes the Riemann zeta function...

zeta function is (usually) a function analogous to the original example, the Riemann zeta function ζ ( s ) = ∑ n = 1 ∞ 1 n s . {\displaystyle \zeta (s)=\sum...

be extended to a meromorphic function defined for all s ≠ 1. The Riemann zeta function is ζ(s,1). The Hurwitz zeta function is named after Adolf Hurwitz...

In mathematics, the Riemann–Siegel theta function is defined in terms of the gamma function as θ ( t ) = arg ⁡ ( Γ ( 1 4 + i t 2 ) ) − log ⁡ π 2 t {\displaystyle...

In mathematics, the prime zeta function is an analogue of the Riemann zeta function, studied by Glaisher (1891). It is defined as the following infinite...

{1}{2^{3}}}+\cdots +{\frac {1}{n^{3}}}\right),\end{aligned}}} where ζ is the Riemann zeta function. It has an approximate value of ζ(3) ≈ 1.2020569031595942853997...

Leonhard Euler proved the Euler product formula for the Riemann zeta function in his thesis Variae observationes circa series infinitas (Various Observations...

and sums over prime powers, introduced by Riemann (1859) for the Riemann zeta function. Such explicit formulae have been applied also to questions on bounding...

continuation elsewhere. In the case when an = n, the zeta function is the ordinary Riemann zeta function. This method was used by Ramanujan to "sum" the series...

initial suggestions of Helmut Hasse and André Weil, motivated by the Riemann zeta function, which results from the case when V is a single point. Taking the...

The von Mangoldt function plays an important role in the theory of Dirichlet series, and in particular, the Riemann zeta function. For example, one has...

Riemann zeta-function on the critical line". Proc. Steklov Inst. Math. (167): 167–178. Selberg, A. (1942). "On the zeros of Riemann's zeta-function"...

expansion of the Riemann zeta function, ζ(s) — and for this reason the Dirichlet eta function is also known as the alternating zeta function, also denoted...

floor of the imaginary part of the first non-trivial zero in the Riemann zeta function is 14 {\displaystyle 14} , in equivalence with its nearest integer...

He gained an international reputation and gave lectures on the Riemann zeta function at universities around the world. Aleksandar Ivić was born in Belgrade...

results on prime numbers (involving the Prime Number Theorem and Riemann zeta function) and additive number theory (such as the Goldbach conjecture and...

including relationships on the Riemann zeta function and the Eisenstein series of modular forms. Divisor functions were studied by Ramanujan, who gave...

{\displaystyle \zeta (s)=s\int _{1}^{\infty }{\frac {\lfloor u\rfloor }{u^{1+s}}}\,du.} where ζ ( s ) {\displaystyle \zeta (s)} is the Riemann zeta function. This...

series, are closely related to the Riemann zeta function, and appear in the expressions of various special functions. The harmonic numbers roughly approximate...

Montgomery (1973) that the pair correlation between pairs of zeros of the Riemann zeta function (normalized to have unit average spacing) is 1 − ( sin ⁡ ( π u )...