In mathematical logic, a theory is complete if it is consistent and for every closed formula in the theory's language, either that formula or its negation...

In model theory, a first-order theory is called model complete if every embedding of its models is an elementary embedding. Equivalently, every first-order...

In computability theory, a system of data-manipulation rules (such as a model of computation, a computer's instruction set, a programming language, or...

satisfiable, such as ω-inconsistent theories. A complete consistent theory (or just a complete theory) is a consistent theory T {\displaystyle {\mathcal {T}}}...

set T {\displaystyle T} . A complete theory is a theory that contains every sentence or its negation. The complete theory of all sentences satisfied by...

modal logics, a logic is structurally complete if every admissible rule is derivable. A theory is model complete if and only if every embedding of its...

In the mathematical area of order theory, completeness properties assert the existence of certain infima or suprema of a given partially ordered set (poset)...

general theories in science and pre-scientific natural philosophy and natural history that have since been superseded by other scientific theories. Many...

provability in first-order logic. The completeness theorem applies to any first-order theory: If T is such a theory, and φ is a sentence (in the same language)...

Complete active space perturbation theory (CASPTn) is a multireference electron correlation method for computational investigation of molecular systems...

In set theory, Zermelo–Fraenkel set theory, named after mathematicians Ernst Zermelo and Abraham Fraenkel, is an axiomatic system that was proposed in...

constants and variables, to form terms. The theory of uninterpreted functions is also sometimes called the free theory, because it is freely generated, and thus...

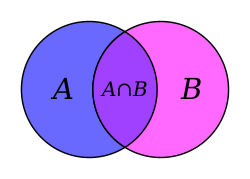
In set theory, the intersection of two sets A {\displaystyle A} and B , {\displaystyle B,} denoted by A ∩ B , {\displaystyle A\cap B,} is the set containing...

In computational complexity theory, a problem is NP-complete when: It is a decision problem, meaning that for any input to the problem, the output is either...

Proof theory is a major branch of mathematical logic and theoretical computer science within which proofs are treated as formal mathematical objects, facilitating...

equivalent as the theory is categorical in κ. Therefore, the theory is complete as all models are equivalent. The assumption that the theory have no finite...

not complete. A consistency proof is a mathematical proof that a particular theory is consistent. The early development of mathematical proof theory was...

Computability theory, also known as recursion theory, is a branch of mathematical logic, computer science, and the theory of computation that originated...

experiment, raised the possibility that quantum mechanics might not be a complete theory. They described two systems physically separated after interacting;...

The propositions of field theory do not concern any one particular application; the mathematician now works in complete abstraction. There are many...

is called complete with respect to a property if every formula with the property can be derived using the axioms. The more general term theory is at times...

In set theory, the complement of a set A, often denoted by A c {\displaystyle A^{c}} (or A′), is the set of elements not in A. When all elements in the...

the sentences of the theory are all true (by the completeness theorem, satisfiability is equivalent to consistency); be complete: for any statement, either...

about limits. The notion of a completed infinity doesn't belong in mathematics." Development of mathematical set theory was motivated by several mathematicians...

In set theory, the union (denoted by ∪) of a collection of sets is the set of all elements in the collection. It is one of the fundamental operations through...

are many "minimal complete set of set-theory operators" that can generate any other set relations. The more popular "Minimal complete operator sets" are...

In physics, a hidden-variable theory is a deterministic model which seeks to explain the probabilistic nature of quantum mechanics by introducing additional...

Major subareas include model theory, proof theory, set theory, and recursion theory (also known as computability theory). Research in mathematical logic...

classes of models in various cardinalities. More precisely, for any complete theory T in a language we write I(T, κ) for the number of models of T (up...

are associated with meanings or semantics. In computational complexity theory, decision problems are typically defined as formal languages, and complexity...