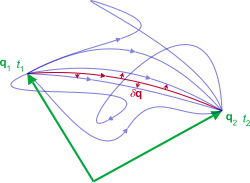
variations and classical mechanics, the Euler–Lagrange equations are a system of second-order ordinary differential equations whose solutions are stationary points...

solving the wave equation. Euler–Lagrange equation, a second-order PDE emerging from minimization problems in calculus of variations. Euler's formula, e ix...

} With these definitions, the Euler–Lagrange equations, or Lagrange's equations of the second kind Lagrange's equations (second kind) d d t ( ∂ L ∂ q...

bending equation: M = − E I d 2 w d x 2 . {\displaystyle M=-EI{d^{2}w \over dx^{2}}.} The dynamic beam equation is the Euler–Lagrange equation for the...

became Senator in 1799. Lagrange was one of the creators of the calculus of variations, deriving the Euler–Lagrange equations for extrema of functionals...

express the Lagrangian as a function on a fiber bundle, wherein the Euler–Lagrange equations can be interpreted as specifying the geodesics on the fiber bundle...

{\boldsymbol {q}})} ⁠, the ( n {\displaystyle n} -dimensional) Euler–Lagrange equation ∂ L ∂ q − d d t ∂ L ∂ q ˙ = 0 {\displaystyle {\frac {\partial {\mathcal...

discovered the three-dimensional wave equation. The Euler–Lagrange equation was developed in the 1750s by Euler and Lagrange in connection with their studies...

Functions that maximize or minimize functionals may be found using the Euler–Lagrange equation of the calculus of variations. A simple example of such a problem...

properties of parabolic equations. See the extensive List of nonlinear partial differential equations. Euler–Lagrange equation Nonlinear system Integrable...

Klein–Gordon equation in physics: φ t t − φ x x + φ = 0. {\displaystyle \varphi _{tt}-\varphi _{xx}+\varphi =0.} The sine-Gordon equation is the Euler–Lagrange equation...

equations of motion for this physical theory should be given by the Euler–Lagrange equations of this functional, which are the Yang–Mills equations derived...

endpoint term adds a boundary condition for the Euler–Lagrange equation (EL-General) which gives the Euler equation with boundary term. Taking the variation...

maximal, one may apply the Euler–Lagrange equation directly, and thus obtain a set of equations equivalent to the geodesic equations. This method has the advantage...

Lagrangian L = T − V {\displaystyle L=T-V} , we can also use Euler–Lagrange equation to solve for equations of motion: ∂ L ∂ x − d d t ( ∂ L ∂ x ˙ ) = 0 {\displaystyle...

to the variational principle are on shell and the Euler–Lagrange equations give the on-shell equations. Noether's theorem regarding differentiable symmetries...

shows that the Euler–Lagrange equations form a n × n {\displaystyle n\times n} system of second-order ordinary differential equations. Inverting the matrix...

perturbations is equivalent to a set of differential equations (called the Euler–Lagrange equations) that may be obtained using the calculus of variations...

δ S = 0 {\displaystyle \delta S=0} is valid if and only if the Euler-Lagrange equations are satisfied, i.e., ∂ L ∂ q k − d d σ ∂ L ∂ q ˙ k = 0 {\displaystyle...

are generated from a Lagrangian density and the field-theoretic Euler–Lagrange equations (see classical field theory for background). In the Schrödinger...

needed] The Leibniz integral rule is used in the derivation of the Euler-Lagrange equation in variational calculus. Differentiation under the integral sign...

dynamics, the Euler equations are a set of partial differential equations governing adiabatic and inviscid flow. They are named after Leonhard Euler. In particular...

differential equations that the system satisfies (e.g., Newton's second law or Euler–Lagrange equations), and sometimes to the solutions to those equations. However...

rather, its (1, ⁠1/2⁠) ⊕ (⁠1/2⁠, 1) part. This field equation can be derived as the Euler–Lagrange equation corresponding to the Rarita–Schwinger Lagrangian:...

conditional upon zero variational derivatives δS/δxλ and leads to Euler–Lagrange equations d d μ ∂ ρ ∂ v λ − ∂ ρ ∂ x λ = 0 , {\displaystyle {\frac {d}{d\mu...

{\mathcal {S}}} is equivalent to a set of differential equations for q(t) (the Euler–Lagrange equations), which may be derived as follows. Let q(t) represent...

partial differential equation L u = 0. {\displaystyle Lu=0.} If the equation L u = 0 {\displaystyle Lu=0} is the Euler–Lagrange equation for some functional...

Beltrami, is a special case of the Euler–Lagrange equation in the calculus of variations. The Euler–Lagrange equation serves to extremize action functionals...

formulated the Euler–Lagrange equation for reducing optimization problems in this area to the solution of differential equations. Euler pioneered the use...

calculus of variations including its most well-known result, the Euler–Lagrange equation. Euler also pioneered the use of analytic methods to solve number theory...