integral calculus, Euler's formula for complex numbers may be used to evaluate integrals involving trigonometric functions. Using Euler's formula, any trigonometric...
5 KB (1,136 words) - 03:08, 20 April 2025
Euler's formula, named after Leonhard Euler, is a mathematical formula in complex analysis that establishes the fundamental relationship between the trigonometric...
26 KB (3,851 words) - 04:01, 16 April 2025
been given simple yet ambiguous names such as Euler's function, Euler's equation, and Euler's formula. Euler's work touched upon so many fields that he is...
15 KB (1,721 words) - 14:18, 9 April 2025
mathematics, the Euler–Maclaurin formula is a formula for the difference between an integral and a closely related sum. It can be used to approximate integrals...
19 KB (3,779 words) - 03:07, 20 April 2025
Gamma function (redirect from Raabe's formula)
and is known as the Euler integral of the second kind. (Euler's integral of the first kind is the beta function.) Using integration by parts, one sees...
90 KB (13,517 words) - 19:06, 28 March 2025
logarithm, also commonly written as ln(x) or loge(x). Euler's constant (sometimes called the Euler–Mascheroni constant) is a mathematical constant, usually...
71 KB (9,583 words) - 02:17, 29 April 2025
Lists of integrals (redirect from Integration formulas)
differencePages displaying wikidata descriptions as a fallback Integration using Euler's formula – Use of complex numbers to evaluate integrals Liouville's theorem...
29 KB (5,610 words) - 14:00, 17 April 2025
has Euler characteristic 2. This viewpoint is implicit in Cauchy's proof of Euler's formula given below. There are many proofs of Euler's formula. One...
29 KB (3,461 words) - 21:33, 8 April 2025
numerical integration of ordinary differential equations and is the simplest Runge–Kutta method. The Euler method is named after Leonhard Euler, who first...
27 KB (4,955 words) - 05:59, 31 January 2025
Verlet integration (French pronunciation: [vɛʁˈlɛ]) is a numerical method used to integrate Newton's equations of motion. It is frequently used to calculate...
28 KB (5,509 words) - 00:40, 12 February 2025
learning resources about Integration by Substitution Integration by substitution at Encyclopedia of Mathematics Area formula at Encyclopedia of Mathematics...
20 KB (3,328 words) - 04:02, 25 April 2025
introduced scientific notation. He discovered what is now known as Euler's formula, that for any real number φ {\displaystyle \varphi } , the complex...
17 KB (2,212 words) - 23:05, 7 April 2025
Lebesgue integral (redirect from Lebesgue integration)
arise in probability theory. The term Lebesgue integration can mean either the general theory of integration of a function with respect to a general measure...
41 KB (5,919 words) - 02:34, 17 March 2025
into an integral by means of the Abel–Plana formula and evaluated using techniques for numerical integration. If the series is truncated at the right time...
10 KB (1,518 words) - 21:17, 14 April 2025
Pick's theorem (redirect from Pick's formula)
using Pick's theorem (proved in a different way) as the basis for a proof of Euler's formula. Alternative proofs of Pick's theorem that do not use Euler's...
20 KB (2,339 words) - 01:48, 17 December 2024
expression that a differential equation is multiplied by to facilitate integration. For example, the nonlinear second order equation d 2 y d t 2 = A y 2...
11 KB (2,623 words) - 13:24, 19 November 2024
Stirling's approximation (redirect from Stirling's formula)
{d}}x=n\ln n-n+1,} and the error in this approximation is given by the Euler–Maclaurin formula: ln ( n ! ) − 1 2 ln n = 1 2 ln 1 + ln 2 + ln 3 + ⋯ +...
26 KB (4,752 words) - 03:10, 20 April 2025
Riemann zeta function (redirect from Euler product formula)
{1}{1-p^{-s}}}\cdots } Both sides of the Euler product formula converge for Re(s) > 1. The proof of Euler's identity uses only the formula for the geometric series and...
74 KB (10,674 words) - 01:04, 20 April 2025
used to find numerical approximations to the solutions of ordinary differential equations (ODEs). Their use is also known as "numerical integration"...
28 KB (3,916 words) - 07:09, 27 January 2025
Riemann integral (redirect from Riemann integration)
theorem of calculus or approximated by numerical integration, or simulated using Monte Carlo integration. Imagine you have a curve on a graph, and the curve...
42 KB (5,479 words) - 01:14, 12 April 2025
Wallis product (redirect from Wallis's formula)
infinite Euler product for π. Wallis sieve The Pippenger product formula obtains e by taking roots of terms in the Wallis product. "Wallis Formula". "Integrating...
9 KB (2,275 words) - 17:59, 8 January 2025
calculus, and more generally in mathematical analysis, integration by parts or partial integration is a process that finds the integral of a product of...
35 KB (6,879 words) - 03:08, 20 April 2025
infinite series. Of course, Euler's original reasoning requires justification (100 years later, Karl Weierstrass proved that Euler's representation of the sine...
44 KB (8,713 words) - 12:19, 31 March 2025
Taylor series (redirect from Taylor formula)
be done readily on the power series representation; for instance, Euler's formula follows from Taylor series expansions for trigonometric and exponential...
48 KB (8,229 words) - 00:43, 11 March 2025
Gaussian integral (redirect from Integration of the normal density function)
e^{-x^{2}}\,dx\right)^{2};} on the other hand, by shell integration (a case of double integration in polar coordinates), its integral is computed to be...
20 KB (4,300 words) - 03:07, 20 April 2025
In mathematics, an Euler–Cauchy equation, or Cauchy–Euler equation, or simply Euler's equation, is a linear homogeneous ordinary differential equation...
12 KB (2,535 words) - 07:18, 21 September 2024
complex analysis, "differentiation is equivalent to integration": complex differentiation, like integration, behaves well under uniform limits – a result that...
25 KB (4,364 words) - 05:30, 12 January 2025
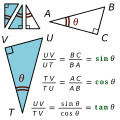
Trigonometric functions (category Use dmy dates from September 2021)
by using above formulas, and then using the identity e a + b = e a e b {\displaystyle e^{a+b}=e^{a}e^{b}} for simplifying the result. Euler's formula can...
77 KB (10,740 words) - 04:00, 13 April 2025
of integration is one of the earliest used. The reduction formula can be derived using any of the common methods of integration, like integration by substitution...
17 KB (2,235 words) - 17:13, 26 March 2024
Fundamental theorem of calculus (category Pages using sidebar with the child parameter)
by symbolic integration, thus avoiding numerical integration. The fundamental theorem of calculus relates differentiation and integration, showing that...
31 KB (4,883 words) - 12:15, 2 May 2025