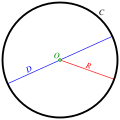
In projective geometry, the harmonic conjugate point of a point on the real projective line with respect to two other points is defined by the following...
16 KB (2,611 words) - 10:07, 13 February 2025
include "harmonic" include: Projective harmonic conjugate Cross-ratio Harmonic analysis Harmonic conjugate Harmonic form Harmonic function Harmonic mean Harmonic...
1 KB (155 words) - 22:09, 14 December 2022
parallelogram with d at the fourth vertex. In projective geometry, the process of finding a projective harmonic conjugate is a ternary operation on three points...
6 KB (708 words) - 23:40, 3 February 2025
throws (German: Wurftheorie). It is based on projective range and the relation of projective harmonic conjugates. Through operations of addition of points...
12 KB (1,614 words) - 01:56, 3 March 2025
Complete quadrangle (category Projective geometry)
at projective harmonic conjugate positions. The four points on the line deriving from the sides and diagonals of the quadrangle are called a harmonic range...
8 KB (942 words) - 05:57, 2 April 2025
Cross-ratio (redirect from Projective invariant)
construction of the projective harmonic conjugate, which he called a throw (German: Wurf): given three points on a line, the harmonic conjugate is a fourth point...
30 KB (4,844 words) - 22:03, 13 May 2025
Under the projective transformations, the incidence structure and the relation of projective harmonic conjugates are preserved. A projective range is the...
38 KB (5,099 words) - 13:20, 23 January 2025
the construction of the projective harmonic conjugate relation between points is part of the structure of the real projective line. For instance, given...
20 KB (3,064 words) - 16:51, 10 August 2023
affine definition of the midpoint M of a segment AB is the projective harmonic conjugate of the point at infinity, P, of the line AB. That is, the point...
11 KB (1,415 words) - 07:36, 15 March 2025
mathematics, the projective line over a ring is an extension of the concept of projective line over a field. Given a ring A (with 1), the projective line P1(A)...
25 KB (2,979 words) - 04:53, 21 March 2025
duality and beyond that to duality in any finite-dimensional projective geometry. A projective plane C may be defined axiomatically as an incidence structure...
47 KB (5,688 words) - 02:41, 24 March 2025
relation of projective harmonic conjugates. Indeed, three points on a projective line determine a fourth by this relation. Application of a projectivity to this...
3 KB (391 words) - 02:24, 10 October 2022
contribution to Feuerbach's theorem. He also made discoveries about projective harmonic conjugates; relating these to the poles and polar lines associated with...
16 KB (1,602 words) - 22:59, 20 December 2024
belonging to arguments θ, θ + π, θ + 2π, θ + 3π the point C is the projective harmonic conjugate of B with respect to A, D, i.e. the cross ratio (A,D;B,C) has...
12 KB (1,373 words) - 18:03, 20 February 2025
In geometry, a real projective line is a projective line over the real numbers. It is an extension of the usual concept of a line that has been historically...
12 KB (1,670 words) - 02:32, 1 December 2024
Conic section (section In the real projective plane)
is the projective harmonic conjugate of the point of intersection of AB and the absolute line, with respect to A and B. A conic in a projective plane that...
69 KB (9,174 words) - 09:13, 17 May 2025
pairs M 1 , M 2 ; E , I {\displaystyle M_{1},M_{2};E,I} are projective harmonic conjugate: Their cross ratio is ( M 1 , M 2 ; E , I ) = − 1 {\displaystyle...
19 KB (3,478 words) - 18:20, 15 February 2025
Poncelet–Steiner theorem (section Projective geometry, as it relates to the Poncelet-Steiner Theorem)
prescribed circle center. This construction is a special case of the projective harmonic conjugate construction, which is not demonstrated in this article. If...
92 KB (12,837 words) - 01:04, 16 May 2025
Hodge theory (redirect from Harmonic form)
complex projective manifold, meaning that X is a closed complex submanifold of some complex projective space CPN. By Chow's theorem, complex projective manifolds...
28 KB (4,339 words) - 19:04, 13 April 2025
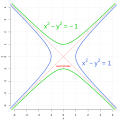
Unit hyperbola (redirect from Quasi-harmonic motion)
interpreted in the projective plane using homogeneous coordinates. Then the asymptotes are lines that are tangent to the projective curve at a point at...
10 KB (1,507 words) - 07:45, 24 April 2025
Pole and polar (category Projective geometry)
line were advanced in projective geometry. For instance, the polar line can be viewed as the set of projective harmonic conjugates of a given point, the...
13 KB (1,358 words) - 15:10, 28 March 2025
"Joachimsthal's Notations". See "Conics and Joachimthal's equation" in Projective harmonic conjugate This article incorporates text from a publication now in the...
2 KB (201 words) - 05:47, 28 March 2025
Point-pair separation (category Projective geometry)
each other. Given any pair of points on a projective line, they separate a third point from its harmonic conjugate. A pair of lines in a pencil separates...
5 KB (601 words) - 02:00, 2 March 2025
corresponds to the process of inversion in projective geometry and taking the projective harmonic conjugate, which can be defined in terms of the cross...
78 KB (10,933 words) - 23:50, 14 April 2025
use of a third parallel midway between the others that is the projective harmonic conjugate of a line at infinity. In a triangle ABC the feet of the altitudes...
6 KB (671 words) - 11:27, 18 July 2024
Centre (geometry) (section Projective conics)
concept of centre in projective geometry uses this relation. The following assertions are from G. B. Halsted. The harmonic conjugate of a point at infinity...
7 KB (923 words) - 23:26, 15 May 2025
Möbius transformation (category Projective geometry)
transformations are the projective transformations of the complex projective line. They form a group called the Möbius group, which is the projective linear group...
70 KB (10,603 words) - 05:07, 10 April 2025
axioms for projective n-space, among other things, he considered the consequences of having a fourth harmonic point be equal to its conjugate. This leads...
10 KB (1,454 words) - 11:02, 9 October 2024
Pappus manipulated the complete quadrangle, used the relation of projective harmonic conjugates, and displayed an awareness of cross-ratios of points and lines...
24 KB (2,983 words) - 04:53, 4 May 2025
(Baker 1922b, vol 2, p. 26) 4. For harmonic conjugate see harmonic. connex A correspondence between a projective space and its dual. consecutive Infinitesimally...
81 KB (11,193 words) - 03:00, 26 December 2024