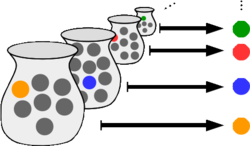
In mathematics, Tychonoff's theorem states that the product of any collection of compact topological spaces is compact with respect to the product topology...

Tikhonov's theorem or Tychonoff's theorem can refer to any of several mathematical theorems named after the Russian mathematician Andrey Nikolayevich Tikhonov:...

space. This version is known as the Schauder–Tychonoff fixed-point theorem. B. V. Singbal proved the theorem for the more general case where K may be non-compact;...

X_{i}} are surjective maps. The axiom of choice is equivalent to Tychonoff's theorem, which states that the product of any collection of compact topological...

)}.} Any product of Hausdorff spaces is again a Hausdorff space. Tychonoff's theorem, which is equivalent to the axiom of choice, states that any product...

topology. As a consequence of Tychonoff's theorem, this product, and hence the unit ball within, is compact. This theorem has applications in physics when...

finitely consistent. The compactness theorem for the propositional calculus is a consequence of Tychonoff's theorem (which says that the product of compact...

compactness theorem and to the Boolean prime ideal theorem) may be used instead. Hahn–Banach can also be proved using Tychonoff's theorem for compact...

that a number of generally accepted mathematical results, such as Tychonoff's theorem, require the axiom of choice for their proofs. Contemporary set theorists...

different method. Despite Tychonoff's article being the first work on the subject of the Stone–Čech compactification and despite Tychonoff's article being referenced...

a consequence of Tychonoff's theorem. A profinite group (e.g. Galois group) is compact. Compactly generated space Compactness theorem Continuous functions...

vector space has a basis, Tychonoff's theorem in topology stating that every product of compact spaces is compact, and the theorems in abstract algebra that...

his work on topology, including the metrization theorem he proved in 1926, and the Tychonoff's theorem, which states that every product of arbitrarily...

limits the maximum feasible production (0 limits the minimum) and Tychonoff's theorem ensures the product of these compacts spaces is compact ensuring...

be a Tychonoff cube (i.e. a possibly infinite product of unit intervals). Every Tychonoff cube is compact Hausdorff as a consequence of Tychonoff's theorem...

the products of compact Hausdorff spaces, since both compactness (Tychonoff's theorem) and the T2 axiom are preserved under arbitrary products. If a normal...

continuous with a compact image, then f has a fixed point. Tikhonov (Tychonoff) fixed-point theorem: Let V be a locally convex topological vector space. For any...

theorem Nowhere dense Baire space Banach–Mazur game Meagre set Comeagre set Compact space Relatively compact subspace Heine–Borel theorem Tychonoff's...

of two paracompact spaces may not be paracompact. Compare this to Tychonoff's theorem, which states that the product of any collection of compact topological...

generalization of the Stone–Weierstrass theorem to noncompact Tychonoff spaces, namely, any continuous function on a Tychonoff space is approximated uniformly...

extension theorem (general topology) Tychonoff's theorem (general topology) Acyclic models theorem (algebraic topology) Blakers–Massey theorem (homotopy...

collections of arbitrary compact spaces. This result is now known as Tychonoff's theorem and is considered one of the most important results in general topology...

mathematical areas like the Hahn–Banach theorem, the Kirszbraun theorem, Tychonoff's theorem, the existence of a Hamel basis for every vector space, and the existence...

Königsberg problem and polyhedron formula are arguably the field's first theorems. The term topology was introduced by Johann Benedict Listing in the 19th...

(f(x))_{x\in X}.} If the codomain Y {\displaystyle Y} is compact, then by Tychonoff's theorem, the space Y X {\displaystyle Y^{X}} is also compact. In measure...

additional axiom must be assumed. Zorn's lemma, the axiom of choice, and Tychonoff's theorem can all be used to prove the ultrafilter lemma. The ultrafilter lemma...

but for finite products they coincide. Related to compactness is Tychonoff's theorem: the (arbitrary) product of compact spaces is compact. Many of these...

particular, equivalent to (a) Zorn's lemma, (b) Tychonoff's theorem, (c) the weak form of the vector basis theorem (which states that every vector space has...

Bruijn–Erdős theorem, by De Bruijn, used transfinite induction. Gottschalk (1951) provided the following very short proof, based on Tychonoff's compactness...

notion of Čech cohomology. He was the first to publish a proof of Tychonoff's theorem in 1937. He was born in Stračov, then in Bohemia, Austria-Hungary...