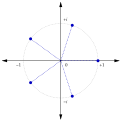
coefficients from an arbitrary field, its reciprocal polynomial or reflected polynomial, denoted by p∗ or pR, is the polynomial p ∗ ( x ) = a n + a n − 1 x + ⋯...

of reciprocal polynomials, when used in CRCs, is that they have exactly the same error-detecting strength as the polynomials they are reciprocals of....

also known as a reciprocal Reciprocal polynomial, a polynomial obtained from another polynomial by reversing its coefficients Reciprocal rule, a technique...

has a root of absolute value 1, the minimal polynomial for a Salem number must be a reciprocal polynomial. This implies that 1 / α {\displaystyle 1/\alpha...

precisely, it is a polynomial function of the coefficients of the original polynomial. The discriminant is widely used in polynomial factoring, number...

and roots of a polynomial Cohn's theorem relating the roots of a self-inversive polynomial with the roots of the reciprocal polynomial of its derivative...

+a_{n}} be a polynomial. The polynomial whose roots are the reciprocals of the roots of P as roots is its reciprocal polynomial Q ( y ) = y n P (...

Q Weil reciprocity law Reciprocal polynomials, the coefficients of the remainder polynomial are the bits of the CRC Reciprocal square root Reciprocity...

multiplicity coincides. Moreover, since the characteristic polynomial of the inverse is the reciprocal polynomial of the original, the eigenvalues share the same...

{1}{z}}} is twice the real part of z. In other words, Φn is a reciprocal polynomial, the polynomial R n {\displaystyle R_{n}} that has r as a root may be deduced...

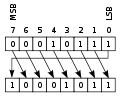
systems get a short check value attached, based on the remainder of a polynomial division of their contents. On retrieval, the calculation is repeated...

resultant of two polynomials is a polynomial expression of their coefficients that is equal to zero if and only if the polynomials have a common root...

the reciprocal polynomial of its derivative. Cohn's theorem is useful for studying the distribution of the roots of self-inversive and self-reciprocal polynomials...

} The associated Narayana polynomial N n ( z ) {\displaystyle {\mathcal {N}}_{n}(z)} is defined as the reciprocal polynomial of N n ( z ) {\displaystyle...

smallest absolute value, one can apply the method on the reciprocal polynomial (polynomial obtained by reversing the order of the coefficients), and...

g(x)h^{*}(x)=x^{n}-1} , where h ∗ ( x ) {\displaystyle h^{*}(x)} denotes the reciprocal polynomial of h ( x ) {\displaystyle h(x)} . The idempotent of C {\displaystyle...

factor is irreducible (but not by Eisenstein's criterion). Only the reciprocal polynomial is irreducible by Eisenstein's criterion. We have now shown that...

The reciprocals of prime numbers have been of interest to mathematicians for various reasons. They do not have a finite sum, as Leonhard Euler proved...

for higher order polynomial equations. If there are more than n + 1 constraints (n being the degree of the polynomial), the polynomial curve can still...

location polynomial. The roots of the error location polynomial can be found by exhaustive search. The error locators Xk are the reciprocals of those...

an algebraic integer. It has minimal polynomial x 2 − x − 1. {\displaystyle x^{2}-x-1.} This quadratic polynomial has two roots, ⁠ φ {\displaystyle \varphi...

complexity that is polynomial in both the size of the input and in the reciprocal of the relative error, 1/η (rather than being polynomial merely in log(1/η)...

investigated orthogonal polynomials (see Laguerre polynomials). Laguerre's method is a root-finding algorithm tailored to polynomials. He laid the foundations...

uses of normalizing constants include making the value of a Legendre polynomial at 1 and in the orthogonality of orthonormal functions. A similar concept...

as a polynomial mod 2. This means that the coefficients of the polynomial must be 1s or 0s. This is called the feedback polynomial or reciprocal characteristic...

"Galois field". In a finite field of order q {\displaystyle q} , the polynomial X q − X {\displaystyle X^{q}-X} has all q {\displaystyle q} elements of...

quadratic polynomial, the only ways to rearrange two roots are to either leave them be or to transpose them, so solving a quadratic polynomial is simple...

an equation that requires the sum of the reciprocals of two positive integers a and b to equal the reciprocal of a third positive integer c: 1 a + 1 b...

algebra, completing the square is a technique for converting a quadratic polynomial of the form ⁠ a x 2 + b x + c {\displaystyle \textstyle ax^{2}+bx+c} ⁠...

mathematics, an Appell sequence, named after Paul Émile Appell, is any polynomial sequence { p n ( x ) } n = 0 , 1 , 2 , … {\displaystyle \{p_{n}(x)\}_{n=0...