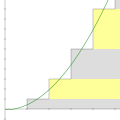
mathematics, analytic continuation is a technique to extend the domain of definition of a given analytic function. Analytic continuation often succeeds...

arithmetic mean of the sequence of partial sums. Other methods involve analytic continuations of related series. In physics, there are a wide variety of summability...

important result about analytic continuation of a complex-analytic function to a larger set. The idea is that one can extend a complex-analytic function (from...

In many-body physics, the problem of analytic continuation is that of numerically extracting the spectral density of a Green function given its values...

zeta function is that it can be defined for other values of s by analytic continuation. One can then define the zeta-regularized sum of 1 + 2 + 3 + 4 +...

The gamma function then is defined in the complex plane as the analytic continuation of this integral function: it is a meromorphic function which is...

an analytic function is a function that is locally given by a convergent power series. There exist both real analytic functions and complex analytic functions...

p-adic analytic manifolds, rigid analytic spaces admit meaningful notions of analytic continuation and connectedness. The basic rigid analytic object...

the principle of analytic continuation which allows extending every real analytic function in a unique way for getting a complex analytic function whose...

analysis, such as holomorphicity, the theory of algebraic curves, and analytic continuation. However, the numerical implementation is rather straightforward...

function is conformal. Analytic continuation is a technique to extend the domain of a given analytic function. Analytic continuation often succeeds in defining...

product expansion, it satisfies a functional equation, it has an analytic continuation to a meromorphic function on the complex plane C with only a simple...

{\displaystyle f(0)=1,} and monotonicity in both argument and modulus. An analytic continuation of Davis' continuous form of the Spiral of Theodorus extends in...

titled "On Integral Equations, Their Solution by Iteration and Analytic Continuation". In 1952, he participated in Project Whirlwind. He joined the faculty...

convergent on a half-plane, that may give rise to an L-function via analytic continuation. The Riemann zeta function is an example of an L-function, and some...

{\displaystyle 1} . It is a special case of a Dirichlet series. By analytic continuation, it can be extended to a meromorphic function on the whole complex...

{\displaystyle z} . Here the branch point is the origin, because the analytic continuation of any solution around a closed loop containing the origin will...

explicit in complex analysis. In the process of analytic continuation, a function that is an analytic function F ( z ) {\displaystyle F(z)} in some open...

differentiable functions) and analytic functions are two very important types of functions. One can easily prove that any analytic function of a real argument...

them that are meromorphic functions of a complex parameter d, the analytic continuation of the number of spacetime dimensions. Dimensional regularization...

{1}{1-z}}} which converges in the larger region Re(z) < 1, giving an analytic continuation of the original series. Considering instead the weak Borel transform...

In physics, a wave packet (also known as a wave train or wave group) is a short burst of localized wave action that travels as a unit, outlined by an envelope...

}}f^{(2k-1)}(x)} where C is a constant specific to the series and its analytic continuation and the limits on the integral were not specified by Ramanujan,...

respect to z at every point of U. Equivalently, it is holomorphic if it is analytic, that is, if its Taylor series exists at every point of U, and converges...

theory. A number of issues were clarified, in particular that of analytic continuation. Here a major difference is evident from the one-variable theory;...

complex functions, typically analytic functions. The domain to which a complex function may be extended by analytic continuation generally consists of almost...

originated in complex analysis, from analytic continuation. It often occurs that one knows the value of a complex analytic function f ( z ) {\displaystyle...

z with |z| < 1; it can be extended to |z| ≥ 1 by the process of analytic continuation. (Here the denominator ks is understood as exp(s ln k)). The special...

\operatorname {Re} (s)>1} , and its analytic continuation elsewhere. The Riemann zeta function plays a pivotal role in analytic number theory and has applications...

such that f ( z ) − R / ( z − a ) {\displaystyle f(z)-R/(z-a)} has an analytic antiderivative in a punctured disk 0 < | z − a | < δ {\displaystyle 0<\vert...